an encyclopedia of finite element definitions
Tiniest tensor H(curl)
Click here to read what the information on this page means.
| Alternative names | TNT H(curl) |
| De Rham complex families | \(\left[S_{3,k}^\square\right]_{1}\) |
| Orders | \(1\leqslant k\) |
| Reference elements | quadrilateral, hexahedron |
| Polynomial set | \(\mathcal{Q}_{k}^d \oplus \mathcal{Z}^{(34)}_{k}\) (quadrilateral) \(\mathcal{Q}_{k}^d \oplus \mathcal{Z}^{(35)}_{k} \oplus \mathcal{Z}^{(36)}_{k} \oplus \mathcal{Z}^{(37)}_{k}\) (hexahedron) ↓ Show polynomial set definitions ↓ |
| DOFs | On each edge: tangent integral moments with an order \(k\) Lagrange space On each face: integral moments with \(\nabla f\times \boldsymbol{n}\) (where \(\boldsymbol{n}\) is a unit vector normal to the face) for each \(f\) in an order \(k\) Lagrange space, and integral moments with \(\nabla\times\boldsymbol{f}\times \boldsymbol{n}\) (where \(\boldsymbol{n}\) is a unit vector normal to the face) for each \(\boldsymbol{f}\) in an order \(k\) vector Lagrange space such that \(\nabla\cdot\boldsymbol{f}=0\) and the normal trace of \(\boldsymbol{f}\) on the edges of the face is 0 On each volume: integral moments with \(\nabla\times\boldsymbol{f}\) for each \(\boldsymbol{f}\) in an order \(k\) vector Lagrange space such that the tangential trace of \(\boldsymbol{f}\) on the faces of the volume is 0, and integral moments with \(\nabla f\) for each \(f\) in an order \(k\) Lagrange space such that the trace of \(f\) on the faces of the volume is 0 |
| Number of DOFs | quadrilateral: \(2(k+1)^2 + 3\) hexahedron: \(3(k+1)^3 + 18\) |
| Mapping | covariant Piola |
| continuity | Components tangential to facets are continuous |
| Categories | Vector-valued elements, H(curl) conforming elements |
Implementations
"TNTcurl"↓ Show Symfem examples ↓ This implementation is used to compute the examples below and verify other implementations. |
Examples
| quadrilateral order 1 | 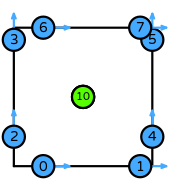 (click to view basis functions) |
| quadrilateral order 2 | 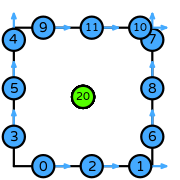 (click to view basis functions) |
| quadrilateral order 3 | 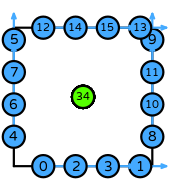 (click to view basis functions) |
| hexahedron order 1 | 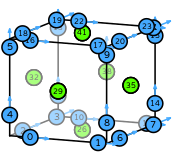 (click to view basis functions) |
References
- Cockburn, Bernardo and Qiu, Weifeng. Commuting diagrams for the TNT elements on cubes, Mathematics of Computation 83, 603–633, 2014. [DOI: 10.1090/S0025-5718-2013-02729-9] [BibTeX]
- Cockburn, Bernardo and Fu, Guosheng. A systematic construction of finite element commuting exact sequences, SIAM journal on numerical analysis 55, 1650–1688, 2017. [DOI: 10.1137/16M1073352] [BibTeX]
DefElement stats
| Element added | 24 October 2021 |
| Element last updated | 16 September 2023 |