an encyclopedia of finite element definitions
Degree 3 Tiniest tensor on a quadrilateral
◀ Back to Tiniest tensor definition page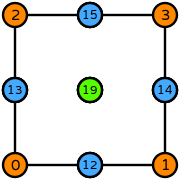
- \(R\) is the reference quadrilateral. The following numbering of the subentities of the reference is used:
- \(\mathcal{V}\) is spanned by: \(1\), \(y\), \(y^{2}\), \(y^{3}\), \(x\), \(x y\), \(x y^{2}\), \(x y^{3}\), \(x^{2}\), \(x^{2} y\), \(x^{2} y^{2}\), \(x^{2} y^{3}\), \(x^{3}\), \(x^{3} y\), \(x^{3} y^{2}\), \(x^{3} y^{3}\), \(\frac{7 x \left(- 5 x^{3} y + 5 x^{3} + 10 x^{2} y - 10 x^{2} - 6 x y + 6 x + y - 1\right)}{3}\), \(\frac{7 x y \left(5 x^{3} - 10 x^{2} + 6 x - 1\right)}{3}\), \(\frac{7 y \left(- 5 x y^{3} + 10 x y^{2} - 6 x y + x + 5 y^{3} - 10 y^{2} + 6 y - 1\right)}{3}\), \(\frac{7 x y \left(5 y^{3} - 10 y^{2} + 6 y - 1\right)}{3}\)
- \(\mathcal{L}=\{l_0,...,l_{19}\}\)
- Functionals and basis functions:
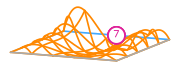
\(\displaystyle l_{0}:v\mapsto v(0,0)\)
\(\displaystyle \phi_{0} = - 35 x^{4} y + 35 x^{4} + 100 x^{3} y^{3} - \frac{4575 x^{3} y^{2}}{26} + \frac{4055 x^{3} y}{26} - 80 x^{3} - \frac{4575 x^{2} y^{3}}{26} + \frac{4035 x^{2} y^{2}}{13} - \frac{5055 x^{2} y}{26} + 60 x^{2} - 35 x y^{4} + \frac{4055 x y^{3}}{26} - \frac{5055 x y^{2}}{26} + \frac{1163 x y}{13} - 16 x + 35 y^{4} - 80 y^{3} + 60 y^{2} - 16 y + 1\)
This DOF is associated with vertex 0 of the reference element.
\(\displaystyle \phi_{0} = - 35 x^{4} y + 35 x^{4} + 100 x^{3} y^{3} - \frac{4575 x^{3} y^{2}}{26} + \frac{4055 x^{3} y}{26} - 80 x^{3} - \frac{4575 x^{2} y^{3}}{26} + \frac{4035 x^{2} y^{2}}{13} - \frac{5055 x^{2} y}{26} + 60 x^{2} - 35 x y^{4} + \frac{4055 x y^{3}}{26} - \frac{5055 x y^{2}}{26} + \frac{1163 x y}{13} - 16 x + 35 y^{4} - 80 y^{3} + 60 y^{2} - 16 y + 1\)
This DOF is associated with vertex 0 of the reference element.
\(\displaystyle l_{1}:v\mapsto v(1,0)\)
\(\displaystyle \phi_{1} = \frac{x \left(- 910 x^{3} y + 910 x^{3} - 2600 x^{2} y^{3} + 4575 x^{2} y^{2} - 415 x^{2} y - 1560 x^{2} + 3225 x y^{3} - 5655 x y^{2} + 1650 x y + 780 x + 910 y^{4} - 2705 y^{3} + 2640 y^{2} - 741 y - 104\right)}{26}\)
This DOF is associated with vertex 1 of the reference element.
\(\displaystyle \phi_{1} = \frac{x \left(- 910 x^{3} y + 910 x^{3} - 2600 x^{2} y^{3} + 4575 x^{2} y^{2} - 415 x^{2} y - 1560 x^{2} + 3225 x y^{3} - 5655 x y^{2} + 1650 x y + 780 x + 910 y^{4} - 2705 y^{3} + 2640 y^{2} - 741 y - 104\right)}{26}\)
This DOF is associated with vertex 1 of the reference element.
\(\displaystyle l_{2}:v\mapsto v(0,1)\)
\(\displaystyle \phi_{2} = \frac{y \left(910 x^{4} - 2600 x^{3} y^{2} + 3225 x^{3} y - 2705 x^{3} + 4575 x^{2} y^{2} - 5655 x^{2} y + 2640 x^{2} - 910 x y^{3} - 415 x y^{2} + 1650 x y - 741 x + 910 y^{3} - 1560 y^{2} + 780 y - 104\right)}{26}\)
This DOF is associated with vertex 2 of the reference element.
\(\displaystyle \phi_{2} = \frac{y \left(910 x^{4} - 2600 x^{3} y^{2} + 3225 x^{3} y - 2705 x^{3} + 4575 x^{2} y^{2} - 5655 x^{2} y + 2640 x^{2} - 910 x y^{3} - 415 x y^{2} + 1650 x y - 741 x + 910 y^{3} - 1560 y^{2} + 780 y - 104\right)}{26}\)
This DOF is associated with vertex 2 of the reference element.
\(\displaystyle l_{3}:v\mapsto v(1,1)\)
\(\displaystyle \phi_{3} = \frac{x y \left(910 x^{3} + 2600 x^{2} y^{2} - 3225 x^{2} y - 935 x^{2} - 3225 x y^{2} + 4020 x y - 15 x + 910 y^{3} - 935 y^{2} - 15 y - 64\right)}{26}\)
This DOF is associated with vertex 3 of the reference element.
\(\displaystyle \phi_{3} = \frac{x y \left(910 x^{3} + 2600 x^{2} y^{2} - 3225 x^{2} y - 935 x^{2} - 3225 x y^{2} + 4020 x y - 15 x + 910 y^{3} - 935 y^{2} - 15 y - 64\right)}{26}\)
This DOF is associated with vertex 3 of the reference element.
\(\displaystyle l_{4}:\mathbf{v}\mapsto\displaystyle\int_{e_{0}}v\)
where \(e_{0}\) is the 0th edge.
\(\displaystyle \phi_{4} = \frac{30 x \left(182 x^{3} y - 182 x^{3} - 221 x^{2} y^{3} + 399 x^{2} y^{2} - 568 x^{2} y + 390 x^{2} + 335 x y^{3} - 607 x y^{2} + 532 x y - 260 x - 114 y^{3} + 208 y^{2} - 146 y + 52\right)}{13}\)
This DOF is associated with edge 0 of the reference element.
where \(e_{0}\) is the 0th edge.
\(\displaystyle \phi_{4} = \frac{30 x \left(182 x^{3} y - 182 x^{3} - 221 x^{2} y^{3} + 399 x^{2} y^{2} - 568 x^{2} y + 390 x^{2} + 335 x y^{3} - 607 x y^{2} + 532 x y - 260 x - 114 y^{3} + 208 y^{2} - 146 y + 52\right)}{13}\)
This DOF is associated with edge 0 of the reference element.
\(\displaystyle l_{5}:\mathbf{v}\mapsto\displaystyle\int_{e_{1}}v\)
where \(e_{1}\) is the 1st edge.
\(\displaystyle \phi_{5} = \frac{30 y \left(- 221 x^{3} y^{2} + 335 x^{3} y - 114 x^{3} + 399 x^{2} y^{2} - 607 x^{2} y + 208 x^{2} + 182 x y^{3} - 568 x y^{2} + 532 x y - 146 x - 182 y^{3} + 390 y^{2} - 260 y + 52\right)}{13}\)
This DOF is associated with edge 1 of the reference element.
where \(e_{1}\) is the 1st edge.
\(\displaystyle \phi_{5} = \frac{30 y \left(- 221 x^{3} y^{2} + 335 x^{3} y - 114 x^{3} + 399 x^{2} y^{2} - 607 x^{2} y + 208 x^{2} + 182 x y^{3} - 568 x y^{2} + 532 x y - 146 x - 182 y^{3} + 390 y^{2} - 260 y + 52\right)}{13}\)
This DOF is associated with edge 1 of the reference element.
\(\displaystyle l_{6}:\mathbf{v}\mapsto\displaystyle\int_{e_{2}}v\)
where \(e_{2}\) is the 2nd edge.
\(\displaystyle \phi_{6} = \frac{30 x y \left(221 x^{2} y^{2} - 335 x^{2} y + 114 x^{2} - 264 x y^{2} + 398 x y - 134 x - 182 y^{3} + 433 y^{2} - 323 y + 72\right)}{13}\)
This DOF is associated with edge 2 of the reference element.
where \(e_{2}\) is the 2nd edge.
\(\displaystyle \phi_{6} = \frac{30 x y \left(221 x^{2} y^{2} - 335 x^{2} y + 114 x^{2} - 264 x y^{2} + 398 x y - 134 x - 182 y^{3} + 433 y^{2} - 323 y + 72\right)}{13}\)
This DOF is associated with edge 2 of the reference element.
\(\displaystyle l_{7}:\mathbf{v}\mapsto\displaystyle\int_{e_{3}}v\)
where \(e_{3}\) is the 3rd edge.
\(\displaystyle \phi_{7} = \frac{30 x y \left(- 182 x^{3} + 221 x^{2} y^{2} - 264 x^{2} y + 433 x^{2} - 335 x y^{2} + 398 x y - 323 x + 114 y^{2} - 134 y + 72\right)}{13}\)
This DOF is associated with edge 3 of the reference element.
where \(e_{3}\) is the 3rd edge.
\(\displaystyle \phi_{7} = \frac{30 x y \left(- 182 x^{3} + 221 x^{2} y^{2} - 264 x^{2} y + 433 x^{2} - 335 x y^{2} + 398 x y - 323 x + 114 y^{2} - 134 y + 72\right)}{13}\)
This DOF is associated with edge 3 of the reference element.
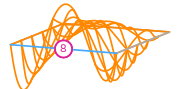
\(\displaystyle l_{8}:\mathbf{v}\mapsto\displaystyle\int_{e_{0}}(2 s_{0})v\)
where \(e_{0}\) is the 0th edge;
and \(s_{0},s_{1}\) is a parametrisation of \(e_{0}\).
\(\displaystyle \phi_{8} = \frac{30 x \left(- 455 x^{3} y + 455 x^{3} + 221 x^{2} y^{3} - 399 x^{2} y^{2} + 1114 x^{2} y - 936 x^{2} - 279 x y^{3} + 510 x y^{2} - 816 x y + 585 x + 58 y^{3} - 111 y^{2} + 157 y - 104\right)}{13}\)
This DOF is associated with edge 0 of the reference element.
where \(e_{0}\) is the 0th edge;
and \(s_{0},s_{1}\) is a parametrisation of \(e_{0}\).
\(\displaystyle \phi_{8} = \frac{30 x \left(- 455 x^{3} y + 455 x^{3} + 221 x^{2} y^{3} - 399 x^{2} y^{2} + 1114 x^{2} y - 936 x^{2} - 279 x y^{3} + 510 x y^{2} - 816 x y + 585 x + 58 y^{3} - 111 y^{2} + 157 y - 104\right)}{13}\)
This DOF is associated with edge 0 of the reference element.
\(\displaystyle l_{9}:\mathbf{v}\mapsto\displaystyle\int_{e_{1}}(2 s_{0})v\)
where \(e_{1}\) is the 1st edge;
and \(s_{0},s_{1}\) is a parametrisation of \(e_{1}\).
\(\displaystyle \phi_{9} = \frac{30 y \left(221 x^{3} y^{2} - 279 x^{3} y + 58 x^{3} - 399 x^{2} y^{2} + 510 x^{2} y - 111 x^{2} - 455 x y^{3} + 1114 x y^{2} - 816 x y + 157 x + 455 y^{3} - 936 y^{2} + 585 y - 104\right)}{13}\)
This DOF is associated with edge 1 of the reference element.
where \(e_{1}\) is the 1st edge;
and \(s_{0},s_{1}\) is a parametrisation of \(e_{1}\).
\(\displaystyle \phi_{9} = \frac{30 y \left(221 x^{3} y^{2} - 279 x^{3} y + 58 x^{3} - 399 x^{2} y^{2} + 510 x^{2} y - 111 x^{2} - 455 x y^{3} + 1114 x y^{2} - 816 x y + 157 x + 455 y^{3} - 936 y^{2} + 585 y - 104\right)}{13}\)
This DOF is associated with edge 1 of the reference element.
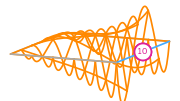
\(\displaystyle l_{10}:\mathbf{v}\mapsto\displaystyle\int_{e_{2}}(2 s_{0})v\)
where \(e_{2}\) is the 2nd edge;
and \(s_{0},s_{1}\) is a parametrisation of \(e_{2}\).
\(\displaystyle \phi_{10} = \frac{30 x y \left(- 221 x^{2} y^{2} + 279 x^{2} y - 58 x^{2} + 264 x y^{2} - 327 x y + 63 x + 455 y^{3} - 979 y^{2} + 633 y - 109\right)}{13}\)
This DOF is associated with edge 2 of the reference element.
where \(e_{2}\) is the 2nd edge;
and \(s_{0},s_{1}\) is a parametrisation of \(e_{2}\).
\(\displaystyle \phi_{10} = \frac{30 x y \left(- 221 x^{2} y^{2} + 279 x^{2} y - 58 x^{2} + 264 x y^{2} - 327 x y + 63 x + 455 y^{3} - 979 y^{2} + 633 y - 109\right)}{13}\)
This DOF is associated with edge 2 of the reference element.
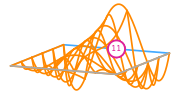
\(\displaystyle l_{11}:\mathbf{v}\mapsto\displaystyle\int_{e_{3}}(2 s_{0})v\)
where \(e_{3}\) is the 3rd edge;
and \(s_{0},s_{1}\) is a parametrisation of \(e_{3}\).
\(\displaystyle \phi_{11} = \frac{30 x y \left(455 x^{3} - 221 x^{2} y^{2} + 264 x^{2} y - 979 x^{2} + 279 x y^{2} - 327 x y + 633 x - 58 y^{2} + 63 y - 109\right)}{13}\)
This DOF is associated with edge 3 of the reference element.
where \(e_{3}\) is the 3rd edge;
and \(s_{0},s_{1}\) is a parametrisation of \(e_{3}\).
\(\displaystyle \phi_{11} = \frac{30 x y \left(455 x^{3} - 221 x^{2} y^{2} + 264 x^{2} y - 979 x^{2} + 279 x y^{2} - 327 x y + 633 x - 58 y^{2} + 63 y - 109\right)}{13}\)
This DOF is associated with edge 3 of the reference element.
\(\displaystyle l_{12}:\mathbf{v}\mapsto\displaystyle\int_{e_{0}}(3 s_{0}^{2})v\)
where \(e_{0}\) is the 0th edge;
and \(s_{0},s_{1}\) is a parametrisation of \(e_{0}\).
\(\displaystyle \phi_{12} = \frac{10 x \left(910 x^{3} y - 910 x^{3} - 1820 x^{2} y + 1820 x^{2} - 105 x y^{3} + 177 x y^{2} + 1020 x y - 1092 x + 105 y^{3} - 177 y^{2} - 110 y + 182\right)}{13}\)
This DOF is associated with edge 0 of the reference element.
where \(e_{0}\) is the 0th edge;
and \(s_{0},s_{1}\) is a parametrisation of \(e_{0}\).
\(\displaystyle \phi_{12} = \frac{10 x \left(910 x^{3} y - 910 x^{3} - 1820 x^{2} y + 1820 x^{2} - 105 x y^{3} + 177 x y^{2} + 1020 x y - 1092 x + 105 y^{3} - 177 y^{2} - 110 y + 182\right)}{13}\)
This DOF is associated with edge 0 of the reference element.
\(\displaystyle l_{13}:\mathbf{v}\mapsto\displaystyle\int_{e_{1}}(3 s_{0}^{2})v\)
where \(e_{1}\) is the 1st edge;
and \(s_{0},s_{1}\) is a parametrisation of \(e_{1}\).
\(\displaystyle \phi_{13} = \frac{10 y \left(- 105 x^{3} y + 105 x^{3} + 177 x^{2} y - 177 x^{2} + 910 x y^{3} - 1820 x y^{2} + 1020 x y - 110 x - 910 y^{3} + 1820 y^{2} - 1092 y + 182\right)}{13}\)
This DOF is associated with edge 1 of the reference element.
where \(e_{1}\) is the 1st edge;
and \(s_{0},s_{1}\) is a parametrisation of \(e_{1}\).
\(\displaystyle \phi_{13} = \frac{10 y \left(- 105 x^{3} y + 105 x^{3} + 177 x^{2} y - 177 x^{2} + 910 x y^{3} - 1820 x y^{2} + 1020 x y - 110 x - 910 y^{3} + 1820 y^{2} - 1092 y + 182\right)}{13}\)
This DOF is associated with edge 1 of the reference element.
\(\displaystyle l_{14}:\mathbf{v}\mapsto\displaystyle\int_{e_{2}}(3 s_{0}^{2})v\)
where \(e_{2}\) is the 2nd edge;
and \(s_{0},s_{1}\) is a parametrisation of \(e_{2}\).
\(\displaystyle \phi_{14} = \frac{10 x y \left(105 x^{2} y - 105 x^{2} - 138 x y + 138 x - 910 y^{3} + 1820 y^{2} - 1059 y + 149\right)}{13}\)
This DOF is associated with edge 2 of the reference element.
where \(e_{2}\) is the 2nd edge;
and \(s_{0},s_{1}\) is a parametrisation of \(e_{2}\).
\(\displaystyle \phi_{14} = \frac{10 x y \left(105 x^{2} y - 105 x^{2} - 138 x y + 138 x - 910 y^{3} + 1820 y^{2} - 1059 y + 149\right)}{13}\)
This DOF is associated with edge 2 of the reference element.
\(\displaystyle l_{15}:\mathbf{v}\mapsto\displaystyle\int_{e_{3}}(3 s_{0}^{2})v\)
where \(e_{3}\) is the 3rd edge;
and \(s_{0},s_{1}\) is a parametrisation of \(e_{3}\).
\(\displaystyle \phi_{15} = \frac{10 x y \left(- 910 x^{3} + 1820 x^{2} + 105 x y^{2} - 138 x y - 1059 x - 105 y^{2} + 138 y + 149\right)}{13}\)
This DOF is associated with edge 3 of the reference element.
where \(e_{3}\) is the 3rd edge;
and \(s_{0},s_{1}\) is a parametrisation of \(e_{3}\).
\(\displaystyle \phi_{15} = \frac{10 x y \left(- 910 x^{3} + 1820 x^{2} + 105 x y^{2} - 138 x y - 1059 x - 105 y^{2} + 138 y + 149\right)}{13}\)
This DOF is associated with edge 3 of the reference element.
\(\displaystyle l_{16}:\mathbf{v}\mapsto\displaystyle\int_{R}(2 s_{0}^{2} - 2 s_{0} + 2 s_{1}^{2} - 2 s_{1})v\)
where \(R\) is the reference element;
and \(s_{0},s_{1}\) is a parametrisation of \(R\).
\(\displaystyle \phi_{16} = \frac{60 x y \left(- 455 x^{2} y^{2} + 735 x^{2} y - 280 x^{2} + 735 x y^{2} - 1191 x y + 456 x - 280 y^{2} + 456 y - 176\right)}{13}\)
This DOF is associated with face 0 of the reference element.
where \(R\) is the reference element;
and \(s_{0},s_{1}\) is a parametrisation of \(R\).
\(\displaystyle \phi_{16} = \frac{60 x y \left(- 455 x^{2} y^{2} + 735 x^{2} y - 280 x^{2} + 735 x y^{2} - 1191 x y + 456 x - 280 y^{2} + 456 y - 176\right)}{13}\)
This DOF is associated with face 0 of the reference element.
\(\displaystyle l_{17}:\mathbf{v}\mapsto\displaystyle\int_{R}(6 s_{0}^{2} s_{1} - 2 s_{0}^{2} - 6 s_{0} s_{1} + 2 s_{0} + 2 s_{1}^{3} - 2 s_{1}^{2})v\)
where \(R\) is the reference element;
and \(s_{0},s_{1}\) is a parametrisation of \(R\).
\(\displaystyle \phi_{17} = \frac{2100 x y \left(26 x^{2} y^{2} - 39 x^{2} y + 13 x^{2} - 42 x y^{2} + 63 x y - 21 x + 16 y^{2} - 24 y + 8\right)}{13}\)
This DOF is associated with face 0 of the reference element.
where \(R\) is the reference element;
and \(s_{0},s_{1}\) is a parametrisation of \(R\).
\(\displaystyle \phi_{17} = \frac{2100 x y \left(26 x^{2} y^{2} - 39 x^{2} y + 13 x^{2} - 42 x y^{2} + 63 x y - 21 x + 16 y^{2} - 24 y + 8\right)}{13}\)
This DOF is associated with face 0 of the reference element.
\(\displaystyle l_{18}:\mathbf{v}\mapsto\displaystyle\int_{R}(2 s_{0}^{3} - 2 s_{0}^{2} + 6 s_{0} s_{1}^{2} - 6 s_{0} s_{1} - 2 s_{1}^{2} + 2 s_{1})v\)
where \(R\) is the reference element;
and \(s_{0},s_{1}\) is a parametrisation of \(R\).
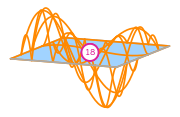
\(\displaystyle \phi_{18} = \frac{2100 x y \left(26 x^{2} y^{2} - 42 x^{2} y + 16 x^{2} - 39 x y^{2} + 63 x y - 24 x + 13 y^{2} - 21 y + 8\right)}{13}\)
This DOF is associated with face 0 of the reference element.
where \(R\) is the reference element;
and \(s_{0},s_{1}\) is a parametrisation of \(R\).
\(\displaystyle \phi_{18} = \frac{2100 x y \left(26 x^{2} y^{2} - 42 x^{2} y + 16 x^{2} - 39 x y^{2} + 63 x y - 24 x + 13 y^{2} - 21 y + 8\right)}{13}\)
This DOF is associated with face 0 of the reference element.
\(\displaystyle l_{19}:\mathbf{v}\mapsto\displaystyle\int_{R}(6 s_{0}^{3} s_{1} - 2 s_{0}^{3} - 6 s_{0}^{2} s_{1} + 2 s_{0}^{2} + 6 s_{0} s_{1}^{3} - 6 s_{0} s_{1}^{2} - 2 s_{1}^{3} + 2 s_{1}^{2})v\)
where \(R\) is the reference element;
and \(s_{0},s_{1}\) is a parametrisation of \(R\).
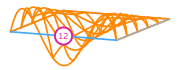
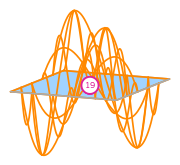
\(\displaystyle \phi_{19} = 2100 x y \left(- 4 x^{2} y^{2} + 6 x^{2} y - 2 x^{2} + 6 x y^{2} - 9 x y + 3 x - 2 y^{2} + 3 y - 1\right)\)
This DOF is associated with face 0 of the reference element.
where \(R\) is the reference element;
and \(s_{0},s_{1}\) is a parametrisation of \(R\).
\(\displaystyle \phi_{19} = 2100 x y \left(- 4 x^{2} y^{2} + 6 x^{2} y - 2 x^{2} + 6 x y^{2} - 9 x y + 3 x - 2 y^{2} + 3 y - 1\right)\)
This DOF is associated with face 0 of the reference element.